Introduction
A table of values shows a list of the values for an independent variable, x, matched with the value of the dependent variable, y. In many cases, an equation can be used to relate the two values. If you know the equation, you can easily generate a table of values.
If you are given the table of values, you must determine how the two values are related and then write an equation that shows the relationship.
|
 |
When looking at the relationship between the y-values and the x-values, it's easy to see that each y-value is 1 more than the x-value. Therefore, the equation that describes this relationship is y = x + 1. |
Develop a Rule When Given a Table of Values
Writing an Equation for a Table of Values
Look at the table below. There does not appear to be a constant rate of change.
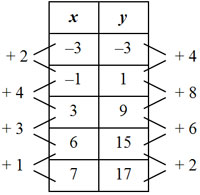
In this example, we look at the ratio of the change in the pattern of y-values and x-values.
Even though there did not appear to be a constant rate of change, there is actually a constant rate of change of 2.
We're almost ready to write our equation.
We now know the value for m (the slope) in the equation y = mx + b is 2. Since m is 2, we know that each x-value is being multiplied by 2.
What else is happening to the x-value to get the corresponding y-value?
| x | Process | y |
| -3 | 2(-3) + 3 | -3 |
| -1 | 2(-1) + 3 | 1 |
| 3 | 2(3) + 3 | 9 |
| 6 | 2(6) + 3 | 15 |
| 7 | 2(7) + 3 | 17 |
We also need to add 3 to the product in order to get the corresponding y-value.
The equation that represents this table of data is y = 2x + 3.
Using a Graphing Calculator to Determine an Equation
Now, we're going to look at how a graphing calculator can help you find an equation that will matches the data in a table. A graphing calculator is needed. If you don't have a graphing calculator, you can use one online here.
Let's look at the following problem. Which of these two equations generates this table of data?
A. y = -4x
B. y = 4x + 8
| x | y |
| -1 | 4 |
| 0 | 8 |
| 1 | 12 |
| 2 | 16 |
We can use the  and
and  features of the graphing calculator to determine which equation is correct.
features of the graphing calculator to determine which equation is correct.
First use  to enter the equation given in answer choice A.
to enter the equation given in answer choice A.
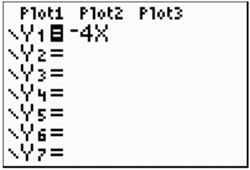
Next use the  function to look at the table of data and compare it to the given table.
function to look at the table of data and compare it to the given table.
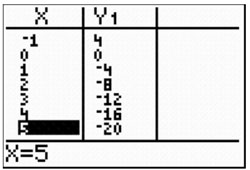
| x | y |
| -1 | -4 |
| 0 | -8 |
| 1 | -12 |
| 2 | -16 |
This set of data is NOT the same as the given table.
(You may need to press 2nd Window to adjust the setup of the table.)
Now, let's check answer choice B.
Enter the equation in 
Use the feature to compare the tables.
feature to compare the tables.
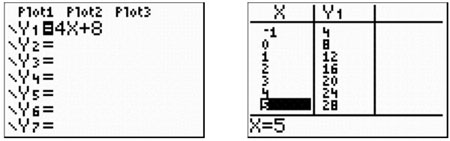
| x | y |
| -1 | 4 |
| 0 | 8 |
| 1 | 12 |
| 2 | 16 |
The data in the table for y = 4x + 8 matches the given table.
Kid2Kid Video
Kid2Kid Video
Chloe has been asked to teach her class about linear and quadratic functions. She is seeking help from Rochelle. Watch the video to see what Rochelle suggests.