Superposition of Waves

Most waves do not look very simple. They look more like the waves in Figure 13.11, rather than the simple water wave considered in the previous sections, which has a perfect sinusoidal shape.

Figure 13.11 These waves result from the superposition of several waves from different sources, producing a complex pattern. (Waterborough, Wikimedia Commons)

Most waves appear complex because they result from two or more simple waves that combine as they come together at the same place at the same time—a phenomenon called superposition.

Waves superimpose by adding their disturbances; each disturbance corresponds to a force, and all the forces add. If the disturbances are along the same line, then the resulting wave is a simple addition of the disturbances of the individual waves, that is, their amplitudes add.

Wave Interference

The two special cases of superposition that produce the simplest results are pure constructive interference and pure destructive interference.

Pure constructive interference occurs when two identical waves arrive at the same point exactly in phase. When waves are exactly in phase, the crests of the two waves are precisely aligned, as are the troughs. Refer to Figure 13.12. Because the disturbances add, the pure constructive interference of two waves with the same amplitude produces a wave that has twice the amplitude of the two individual waves, but has the same wavelength.

Figure 13.12 The pure constructive interference of two identical waves produces a wave with twice the amplitude but the same wavelength.

Figure 13.13 shows two identical waves that arrive exactly out of phase—that is, precisely aligned crest to trough—producing pure destructive interference. Because the disturbances are in opposite directions for this superposition, the resulting amplitude is zero for pure destructive interference; that is, the waves completely cancel out each other.

Figure 13.13 The pure destructive interference of two identical waves produces zero amplitude, or complete cancellation.

While pure constructive interference and pure destructive interference can occur, they are not very common because they require precisely aligned identical waves. The superposition of most waves that we see in nature produces a combination of constructive and destructive interferences.

Waves that are not results of pure constructive or destructive interference can vary from place to place and time to time. The sound from a stereo, for example, can be loud in one spot and soft in another. The varying loudness means that the sound waves add partially constructively and partially destructively at different locations. A stereo has at least two speakers that create sound waves, and waves can reflect from walls. All these waves superimpose.

An example of sounds that vary over time from constructive to destructive is found in the combined whine of jet engines heard by a stationary passenger. The volume of the combined sound can fluctuate up and down as the sound from the two engines varies in time from constructive to destructive.

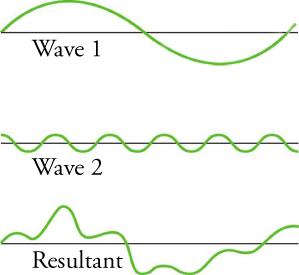
The two previous examples considered waves that are similar—both stereo speakers generate sound waves with the same amplitude and wavelength, as do the jet engines. But what happens when two waves that are not similar, that is, having different amplitudes and wavelengths, are superimposed? An example of the superposition of two dissimilar waves is shown in Figure 13.14. Here again, the disturbances add and subtract, but they produce an even more complicated-looking wave. The resultant wave from the combined disturbances of two dissimilar waves looks much different than the idealized sinusoidal shape of a periodic wave.

Figure 13.14 The superposition of nonidentical waves exhibits both constructive and destructive interferences.

Virtual Physics

Wave Interference

Figure 13.15 Click here for the Wave Interference simulation.

In this simulation, make waves with a dripping faucet, an audio speaker, or a laser by switching between the water, sound, and light tabs. Contrast and compare how the different types of waves behave. Try rotating the view from top to side to make observations. Then experiment with adding a second source or a pair of slits to create an interference pattern.

Grasp Check

In the water tab, compare the waves generated by one drip versus two drips. What happens to the amplitude of the waves when there are two drips? Is this constructive or destructive interference? Why would this be the case?

1. The amplitude of the water waves remains same because of the destructive interference as the drips of water hit the surface at the same time.
2. The amplitude of the water waves is canceled because of the destructive interference as the drips of water hit the surface at the same time.
3. The amplitude of water waves remains same because of the constructive interference as the drips of water hit the surface at the same time.
4. The amplitude of water waves doubles because of the constructive interference as the drips of water hit the surface at the same time.

Standing Waves

Sometimes waves do not seem to move and they appear to just stand in place, vibrating. Such waves are called standing waves and are formed by the superposition of two or more waves moving in opposite directions. The waves move through each other with their disturbances adding as they go by. If the two waves have the same amplitude and wavelength, then they alternate between constructive and destructive interference. Standing waves created by the superposition of two identical waves moving in opposite directions are illustrated in Figure 13.16.

Figure 13.16 A standing wave is created by the superposition of two identical waves moving in opposite directions. The oscillations are at fixed locations in space and result from alternating constructive and destructive interferences.

As an example, standing waves can be seen on the surface of a glass of milk in a refrigerator. The vibrations from the refrigerator motor create waves on the milk that oscillate up and down but do not seem to move across the surface. The two waves that produce standing waves may be due to the reflections from the side of the glass.

Earthquakes can create standing waves and cause constructive and destructive interferences. As the earthquake waves travel along the surface of Earth and reflect off denser rocks, constructive interference occurs at certain points. As a result, areas closer to the epicenter are not damaged while areas farther from the epicenter are damaged.

Standing waves are also found on the strings of musical instruments and are due to reflections of waves from the ends of the string. Figure 13.17 and Figure 13.18 show three standing waves that can be created on a string that is fixed at both ends. When the wave reaches the fixed end, it has nowhere else to go but back where it came from, causing the reflection. The nodes are the points where the string does not move; more generally, the nodes are the points where the wave disturbance is zero in a standing wave. The fixed ends of strings must be nodes, too, because the string cannot move there.

The antinode is the location of maximum amplitude in standing waves. The standing waves on a string have a frequency that is related to the propagation speed $v_w$ of the disturbance on the string. The wavelength $\lambda$ is determined by the distance between the points where the string is fixed in place.

Figure 13.17 The figure shows a string oscillating with its maximum disturbance as the antinode.

Figure 13.18 The figure shows a string oscillating with multiple nodes.

Reflection and Refraction of Waves

As we saw in the case of standing waves on the strings of a musical instrument, reflection is the change in direction of a wave when it bounces off a barrier, such as a fixed end. When the wave hits the fixed end, it changes direction, returning to its source. As it is reflected, the wave experiences an inversion, which means that it flips vertically. If a wave hits the fixed end with a crest, it will return as a trough, and vice versa (Henderson 2015). Refer to Figure 13.19.

Figure 13.19 A wave is inverted after reflection from a fixed end.

Rather than encountering a fixed end or barrier, waves sometimes pass from one medium into another, for instance, from air into water. Different types of media have different properties, such as density or depth, that affect how a wave travels through them. At the boundary between media, waves experience refraction—they change their path of propagation. As the wave bends, it also changes its speed and wavelength upon entering the new medium. Refer to Figure 13.20.

Figure 13.20 A wave refracts as it enters a different medium.

For example, water waves traveling from the deep end to the shallow end of a swimming pool experience refraction. They bend in a path closer to perpendicular to the surface of the water, propagate slower, and decrease in wavelength as they enter shallower water.